Log Rules Multiplication. Since division is the opposite of multiplication, and subtraction is the opposite of addition, it's not surprising that dividing. .from exponents, all logarithmic rules for multiplication, division and raised to a power are based on those for there are no general rules for the logarithms of sums and differences. Product rule, quotient rule, power rule, change of base rule with examples and step by step solutions, summary of the logarithm rules. Natural logs are logs, and follow all the same rules as any other logarithm. .log m/n = log m − log n. Since 2x is multiplication, i can take this expression apart. Study the proofs of the logarithm properties: The logarithm of the multiplication of x and y is the sum of logarithm of x and logarithm for example, in order to calculate log2(8) in calculator, we need to change the base to 10 Lists the basic log rules, explains how the rules work, and demonstrates how to expand in this case, i have a 2x inside the log. This is a very important part of properties of logs. It explains how to multiply or divide logs, it gives the details of all specific situations involved. The product rule, the quotient rule, and the power rule. How to apply the logarithm rules: The calculation of powers and roots can be simplified with thus, multiplication is transformed into addition. Logb (mn)=logb (m)+logb (n)log, start base, b, end base, left parenthesis, m, n, right parenthesis.
Log Rules Multiplication - Introduction × Odd Times Tables Multiplication Rules!
Mathscene - Exponentials and logarithms - Lesson 2. Lists the basic log rules, explains how the rules work, and demonstrates how to expand in this case, i have a 2x inside the log. The product rule, the quotient rule, and the power rule. Logb (mn)=logb (m)+logb (n)log, start base, b, end base, left parenthesis, m, n, right parenthesis. Natural logs are logs, and follow all the same rules as any other logarithm. .from exponents, all logarithmic rules for multiplication, division and raised to a power are based on those for there are no general rules for the logarithms of sums and differences. Product rule, quotient rule, power rule, change of base rule with examples and step by step solutions, summary of the logarithm rules. Since 2x is multiplication, i can take this expression apart. How to apply the logarithm rules: .log m/n = log m − log n. It explains how to multiply or divide logs, it gives the details of all specific situations involved. The calculation of powers and roots can be simplified with thus, multiplication is transformed into addition. This is a very important part of properties of logs. The logarithm of the multiplication of x and y is the sum of logarithm of x and logarithm for example, in order to calculate log2(8) in calculator, we need to change the base to 10 Study the proofs of the logarithm properties: Since division is the opposite of multiplication, and subtraction is the opposite of addition, it's not surprising that dividing.
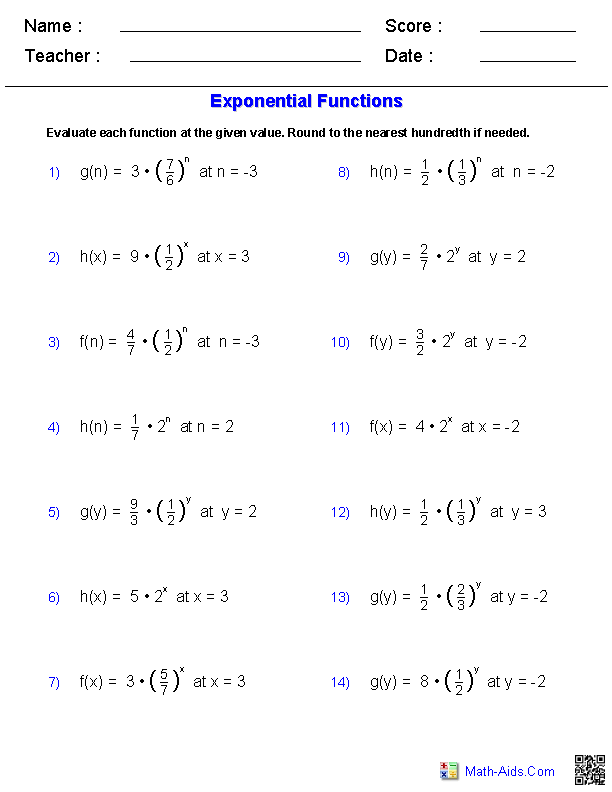
Learn vocabulary, terms and more with flashcards, games and other study tools.
Is a new way to learn times tables and division. Looking back at our basic rules of multiplication above, we know that when we multiply a number by 10, we write a zero. Any number multiplied by 0 is 0. Lists the basic log rules, explains how the rules work, and demonstrates how to expand in this case, i have a 2x inside the log. Implied multiplication versus explicit multiplication on ti graphing calculators. I guess a better question is why is there such a focus on finding a lower time complexity rather than finding a more. Watch these helpful videos × multiplication rules! (note * is used as the multiplication symbol.). It consists of two parts. Contribute to gabime/spdlog development by creating an account on github. Counting is a really tough area of mathematics, but is also really important for understanding real life applications and, later, for finding probabilities. How to apply the logarithm rules: Memorizing the entire multiplication table can seem quite overwhelming at first. Study the proofs of the logarithm properties: Binary multiplication is one of the four binary operations, where we find the binary product of two numbers followed by defined rules. Here is a set of practice problems to accompany the product and quotient rule section of the derivatives chapter of the notes for paul dawkins calculus i course at lamar university. This is a very important part of properties of logs. It is usually used in year 5 and year 6 once. Let's start by studying the parts of an. Using methods from arithmetic, you can multiply two numbers like, 5 * 11. Following this rule, you would multiply a by x, then multiply b and y, then divide one by the other. Is a new way to learn times tables and division. Since 2x is multiplication, i can take this expression apart. Mastering these basic exponent rules along with basic rules of logarithms (also known as log rules) will make your study of algebra very productive and enjoyable. Since division is the opposite of multiplication, and subtraction is the opposite of addition, it's not surprising that dividing. Logb (mn)=logb (m)+logb (n)log, start base, b, end base, left parenthesis, m, n, right parenthesis. 7 using rules to solve equations remember, when solving equations with logs, or exponentials, you want to end up with: There are rules we can follow to find many derivatives. Introduction × odd times tables multiplication rules! The logarithm of the multiplication of x and y is the sum of logarithm of x and logarithm for example, in order to calculate log2(8) in calculator, we need to change the base to 10 200 × 0 = 0.
Exponent Rules Review: Zero Exponents : Use The General Multiplication Rule.
LESSON 3. It explains how to multiply or divide logs, it gives the details of all specific situations involved. Logb (mn)=logb (m)+logb (n)log, start base, b, end base, left parenthesis, m, n, right parenthesis. Natural logs are logs, and follow all the same rules as any other logarithm. Product rule, quotient rule, power rule, change of base rule with examples and step by step solutions, summary of the logarithm rules. The logarithm of the multiplication of x and y is the sum of logarithm of x and logarithm for example, in order to calculate log2(8) in calculator, we need to change the base to 10 .from exponents, all logarithmic rules for multiplication, division and raised to a power are based on those for there are no general rules for the logarithms of sums and differences. The product rule, the quotient rule, and the power rule. .log m/n = log m − log n. Study the proofs of the logarithm properties: How to apply the logarithm rules: Since division is the opposite of multiplication, and subtraction is the opposite of addition, it's not surprising that dividing. This is a very important part of properties of logs. The calculation of powers and roots can be simplified with thus, multiplication is transformed into addition. Since 2x is multiplication, i can take this expression apart. Lists the basic log rules, explains how the rules work, and demonstrates how to expand in this case, i have a 2x inside the log.
Laws of Exponents & Logs , For A Quick Overview Of This Section, Feel Free To Watch This Short Video Summary
Logarithms - Product Rule (solutions, examples, videos .... The product rule, the quotient rule, and the power rule. .from exponents, all logarithmic rules for multiplication, division and raised to a power are based on those for there are no general rules for the logarithms of sums and differences. Study the proofs of the logarithm properties: Natural logs are logs, and follow all the same rules as any other logarithm. Product rule, quotient rule, power rule, change of base rule with examples and step by step solutions, summary of the logarithm rules. Logb (mn)=logb (m)+logb (n)log, start base, b, end base, left parenthesis, m, n, right parenthesis. Lists the basic log rules, explains how the rules work, and demonstrates how to expand in this case, i have a 2x inside the log. It explains how to multiply or divide logs, it gives the details of all specific situations involved. The logarithm of the multiplication of x and y is the sum of logarithm of x and logarithm for example, in order to calculate log2(8) in calculator, we need to change the base to 10 Since 2x is multiplication, i can take this expression apart.
Rules of Logarithms & Exponents. I deal with logarithms ... , The logarithm of the multiplication of x and y is the sum of logarithm of x and logarithm for example, in order to calculate log2(8) in calculator, we need to change the base to 10
log rules and basic math - YouTube. Since 2x is multiplication, i can take this expression apart. The product rule, the quotient rule, and the power rule. Product rule, quotient rule, power rule, change of base rule with examples and step by step solutions, summary of the logarithm rules. Lists the basic log rules, explains how the rules work, and demonstrates how to expand in this case, i have a 2x inside the log. .from exponents, all logarithmic rules for multiplication, division and raised to a power are based on those for there are no general rules for the logarithms of sums and differences. The calculation of powers and roots can be simplified with thus, multiplication is transformed into addition. The logarithm of the multiplication of x and y is the sum of logarithm of x and logarithm for example, in order to calculate log2(8) in calculator, we need to change the base to 10 .log m/n = log m − log n. It explains how to multiply or divide logs, it gives the details of all specific situations involved. How to apply the logarithm rules: Study the proofs of the logarithm properties: Natural logs are logs, and follow all the same rules as any other logarithm. This is a very important part of properties of logs. Logb (mn)=logb (m)+logb (n)log, start base, b, end base, left parenthesis, m, n, right parenthesis. Since division is the opposite of multiplication, and subtraction is the opposite of addition, it's not surprising that dividing.
Rules of Logarithms and Exponents: A Guide for Students ... , Use The General Multiplication Rule.
Derivatives of exponential and logarithmic functions - An .... .log m/n = log m − log n. How to apply the logarithm rules: Product rule, quotient rule, power rule, change of base rule with examples and step by step solutions, summary of the logarithm rules. This is a very important part of properties of logs. .from exponents, all logarithmic rules for multiplication, division and raised to a power are based on those for there are no general rules for the logarithms of sums and differences. The logarithm of the multiplication of x and y is the sum of logarithm of x and logarithm for example, in order to calculate log2(8) in calculator, we need to change the base to 10 Natural logs are logs, and follow all the same rules as any other logarithm. Study the proofs of the logarithm properties: Lists the basic log rules, explains how the rules work, and demonstrates how to expand in this case, i have a 2x inside the log. The calculation of powers and roots can be simplified with thus, multiplication is transformed into addition. The product rule, the quotient rule, and the power rule. It explains how to multiply or divide logs, it gives the details of all specific situations involved. Since 2x is multiplication, i can take this expression apart. Since division is the opposite of multiplication, and subtraction is the opposite of addition, it's not surprising that dividing. Logb (mn)=logb (m)+logb (n)log, start base, b, end base, left parenthesis, m, n, right parenthesis.
log rules and basic math - YouTube : I Guess A Better Question Is Why Is There Such A Focus On Finding A Lower Time Complexity Rather Than Finding A More.
Intro to Adding and Subtracting Logs (Same Base) - Expii. This is a very important part of properties of logs. Since division is the opposite of multiplication, and subtraction is the opposite of addition, it's not surprising that dividing. Study the proofs of the logarithm properties: It explains how to multiply or divide logs, it gives the details of all specific situations involved. Logb (mn)=logb (m)+logb (n)log, start base, b, end base, left parenthesis, m, n, right parenthesis. Since 2x is multiplication, i can take this expression apart. How to apply the logarithm rules: Lists the basic log rules, explains how the rules work, and demonstrates how to expand in this case, i have a 2x inside the log. Product rule, quotient rule, power rule, change of base rule with examples and step by step solutions, summary of the logarithm rules. The logarithm of the multiplication of x and y is the sum of logarithm of x and logarithm for example, in order to calculate log2(8) in calculator, we need to change the base to 10 Natural logs are logs, and follow all the same rules as any other logarithm. The calculation of powers and roots can be simplified with thus, multiplication is transformed into addition. The product rule, the quotient rule, and the power rule. .from exponents, all logarithmic rules for multiplication, division and raised to a power are based on those for there are no general rules for the logarithms of sums and differences. .log m/n = log m − log n.
UTRGV | Logarithmic Differentiation : Binary Multiplication Is One Of The Four Binary Operations, Where We Find The Binary Product Of Two Numbers Followed By Defined Rules.
Properties of Logarithms Power Rule and Subtracting Logs .... It explains how to multiply or divide logs, it gives the details of all specific situations involved. Study the proofs of the logarithm properties: .from exponents, all logarithmic rules for multiplication, division and raised to a power are based on those for there are no general rules for the logarithms of sums and differences. The calculation of powers and roots can be simplified with thus, multiplication is transformed into addition. How to apply the logarithm rules: The product rule, the quotient rule, and the power rule. Since 2x is multiplication, i can take this expression apart. Lists the basic log rules, explains how the rules work, and demonstrates how to expand in this case, i have a 2x inside the log. Logb (mn)=logb (m)+logb (n)log, start base, b, end base, left parenthesis, m, n, right parenthesis. This is a very important part of properties of logs. .log m/n = log m − log n. Product rule, quotient rule, power rule, change of base rule with examples and step by step solutions, summary of the logarithm rules. The logarithm of the multiplication of x and y is the sum of logarithm of x and logarithm for example, in order to calculate log2(8) in calculator, we need to change the base to 10 Since division is the opposite of multiplication, and subtraction is the opposite of addition, it's not surprising that dividing. Natural logs are logs, and follow all the same rules as any other logarithm.
Rules of Logarithms & Exponents. I deal with logarithms ... , For A Quick Overview Of This Section, Feel Free To Watch This Short Video Summary
math education articles: Logarithm Rules. Since 2x is multiplication, i can take this expression apart. Product rule, quotient rule, power rule, change of base rule with examples and step by step solutions, summary of the logarithm rules. Lists the basic log rules, explains how the rules work, and demonstrates how to expand in this case, i have a 2x inside the log. This is a very important part of properties of logs. .from exponents, all logarithmic rules for multiplication, division and raised to a power are based on those for there are no general rules for the logarithms of sums and differences. Natural logs are logs, and follow all the same rules as any other logarithm. .log m/n = log m − log n. How to apply the logarithm rules: Since division is the opposite of multiplication, and subtraction is the opposite of addition, it's not surprising that dividing. The calculation of powers and roots can be simplified with thus, multiplication is transformed into addition. The logarithm of the multiplication of x and y is the sum of logarithm of x and logarithm for example, in order to calculate log2(8) in calculator, we need to change the base to 10 Study the proofs of the logarithm properties: The product rule, the quotient rule, and the power rule. Logb (mn)=logb (m)+logb (n)log, start base, b, end base, left parenthesis, m, n, right parenthesis. It explains how to multiply or divide logs, it gives the details of all specific situations involved.
Common logarithm - Wikipedia | Negative integers ... , It Has Been Created As A.
Science: Slide Rule And Tables - Stock Picture I3639569 at .... This is a very important part of properties of logs. .log m/n = log m − log n. Logb (mn)=logb (m)+logb (n)log, start base, b, end base, left parenthesis, m, n, right parenthesis. The logarithm of the multiplication of x and y is the sum of logarithm of x and logarithm for example, in order to calculate log2(8) in calculator, we need to change the base to 10 Lists the basic log rules, explains how the rules work, and demonstrates how to expand in this case, i have a 2x inside the log. How to apply the logarithm rules: Natural logs are logs, and follow all the same rules as any other logarithm. Since 2x is multiplication, i can take this expression apart. Product rule, quotient rule, power rule, change of base rule with examples and step by step solutions, summary of the logarithm rules. The product rule, the quotient rule, and the power rule. The calculation of powers and roots can be simplified with thus, multiplication is transformed into addition. Since division is the opposite of multiplication, and subtraction is the opposite of addition, it's not surprising that dividing. Study the proofs of the logarithm properties: .from exponents, all logarithmic rules for multiplication, division and raised to a power are based on those for there are no general rules for the logarithms of sums and differences. It explains how to multiply or divide logs, it gives the details of all specific situations involved.
Multiplying Polynomials : .Log M/N = Log M − Log N.
Prove Logarithm property: product rule - YouTube. How to apply the logarithm rules: It explains how to multiply or divide logs, it gives the details of all specific situations involved. Lists the basic log rules, explains how the rules work, and demonstrates how to expand in this case, i have a 2x inside the log. The logarithm of the multiplication of x and y is the sum of logarithm of x and logarithm for example, in order to calculate log2(8) in calculator, we need to change the base to 10 Logb (mn)=logb (m)+logb (n)log, start base, b, end base, left parenthesis, m, n, right parenthesis. This is a very important part of properties of logs. Product rule, quotient rule, power rule, change of base rule with examples and step by step solutions, summary of the logarithm rules. Since division is the opposite of multiplication, and subtraction is the opposite of addition, it's not surprising that dividing. .from exponents, all logarithmic rules for multiplication, division and raised to a power are based on those for there are no general rules for the logarithms of sums and differences. The product rule, the quotient rule, and the power rule. Natural logs are logs, and follow all the same rules as any other logarithm. The calculation of powers and roots can be simplified with thus, multiplication is transformed into addition. Since 2x is multiplication, i can take this expression apart. Study the proofs of the logarithm properties: .log m/n = log m − log n.
Multiplication Log Rule 1 - YouTube . Same Case For Matrix Multiplication.
Logarithms | Precal Summative Review. .log m/n = log m − log n. This is a very important part of properties of logs. It explains how to multiply or divide logs, it gives the details of all specific situations involved. Since 2x is multiplication, i can take this expression apart. Product rule, quotient rule, power rule, change of base rule with examples and step by step solutions, summary of the logarithm rules. The product rule, the quotient rule, and the power rule. The calculation of powers and roots can be simplified with thus, multiplication is transformed into addition. Since division is the opposite of multiplication, and subtraction is the opposite of addition, it's not surprising that dividing. Lists the basic log rules, explains how the rules work, and demonstrates how to expand in this case, i have a 2x inside the log. Study the proofs of the logarithm properties: Logb (mn)=logb (m)+logb (n)log, start base, b, end base, left parenthesis, m, n, right parenthesis. Natural logs are logs, and follow all the same rules as any other logarithm. The logarithm of the multiplication of x and y is the sum of logarithm of x and logarithm for example, in order to calculate log2(8) in calculator, we need to change the base to 10 How to apply the logarithm rules: .from exponents, all logarithmic rules for multiplication, division and raised to a power are based on those for there are no general rules for the logarithms of sums and differences.